Table of Contents
ToggleBack EMF in DC generator
In generator, when a conductor cuts the magnetic flux, an EMF gets induced in the conductor. In the motor, after motoring action, armature starts rotating & armature conductor cuts the flux. So there exists a generating action in motor. Due to this generating action, EMF will be induced in the armature conductors. This induced EMF always acts in the opposite direction to the supply voltage according to Lenz’s law.
In a DC motor, electrical input is converted in to mechanical output. Hence the EMF induced opposes the supply voltage. This EMF tries to set up a current in the armature which is in the opposite direction to that of supply voltage. Hence it is known as back EMF and denoted by Eb. The magnitude of Eb is same as that of generator as it is generated by generating action.
Eb = ǾZNP/60A Volts
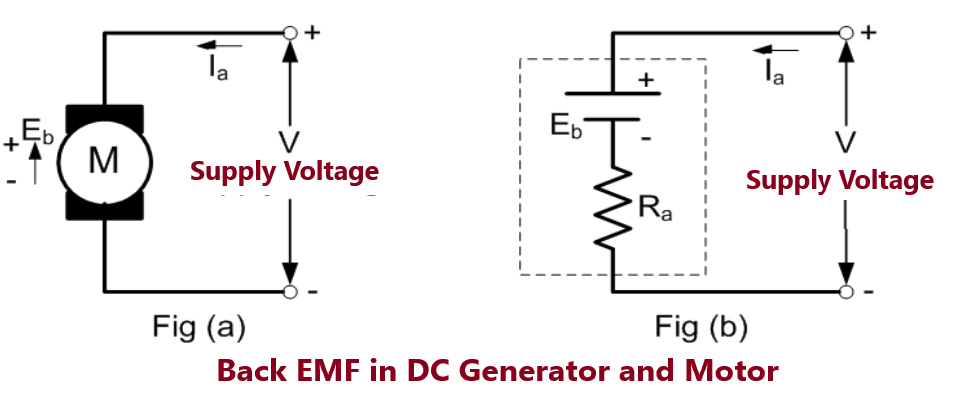
This EMF is shown schematically in fig (a). If V is the supply voltage in volts, & Ra is the armature resistance, the equivalent circuit can be as shown in fig.B.
Significance of Back EMF
Voltage equation of a DC motor
In motor, the supply voltage V has to overcome back EMF Eb which opposes V and also various drops of the armature and brushes etc. Hence voltage equation of a DC motor can be written as:
V=Eb+IaRa+Brush Drop
V=Eb+IaRa , neglecting brush drop
The back EMF Eb is always less than the supply voltage V. Hence armature current is decided by the difference of V &Eb can be written as
Ia=(V-Eb)/Ra Amps
So back EMF regulates the flow of armature current and it automatically alters the armature current to meet the load requirements. Due to the presence of back EMF, the DC motor becomes a regulating machine.
As the mechanical load on the DC motor increases, the speed of the motor reduces which in turn increases the difference of V &Eb there by motor draws more current from the supply and vice versa when the load decreases.
Power equation of a DC motor
The voltage equation of a DC motor is given by
V=Eb+IaRa , Multiplying both sides by Ia, we get
VIa=EbIa+Ia2Ra
This is the power equation of the DC motor.
Where V Ia Net electrical input to armature in watts
Ia2Ra Power loss due to resistance of armature called armature copper loss
Difference between these two i.e VIa– Ia2Ra gives the output of the armature. So EbIa is called the electrical equivalent of gross mechanical power developed by the armature. This is denoted by Pm. Therefore,
Pm=EbIa=VIa– Ia2Ra
Principle of DC Motors
When a current carrying conductor is placed in a magnetic field, it experiences a mechanical force whose direction is given by Fleming’s left hand rule & magnitude is given by:
F=B.I.l Newtons
B – Flux Density in Wb / m2
I – Current through conductor in Amperes
l- Length of Conductor in mtrs
In a practical DC motor, magnetic field is produced by electromagnets while the armature conductors play a role of current carrying conductors and hence armature conductors experience a force. The force experienced by individual conductors acts as a twisting or turning force on the armature which is called a torque. The torque is the product of force and the radius at which this force acts. So overall armature experiences a torque and starts rotating.

Pingback: Three-point starter method of DC Motor
Pingback: Interview question on DC Machine